What is the filter-based characterization of continuity in topological spaces?
Reference

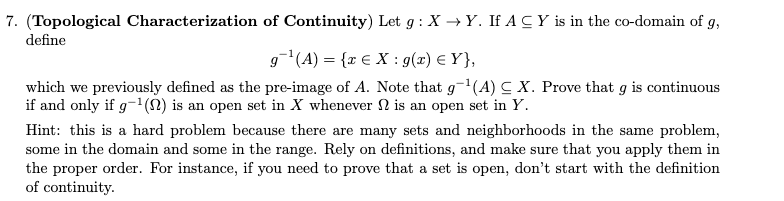

OpenAI's Answer
In topology, the filter-based characterization of continuity involves the concept of filters. Filters are collections of sets that generalize notions like sequences and neighborhoods.
Definition:
A function ( f: X \to Y ) between two topological spaces is continuous if for every filter (\mathcal{F}) on (X) that converges to a point (x \in X), the filter (f(\mathcal{F})) on (Y) converges to (f(x)).
Filters in Topology:
- Filters: A filter is a non-empty collection of subsets of a set that is closed under finite intersections and supersets.
- Neighborhood Filter: For a point (x \in X), the neighborhood filter consists of all neighborhoods of (x).
This approach using filters provides an alternative to the usual open-set definition and is particularly useful in the study of convergence and continuity in more abstract settings.
For more detailed information, you can refer to the Wikipedia article on Filters in topology.